An Overview
Cosmology examines the entire Universe as a closed system and examines its evolution. What do we currently know about the Universe? Here is a short overview:
The Universe is homogeneous and isotropic
Since Copernicus, the idea that there are special places or directions in the Universe is disfavoured. Homogeneity, i.e. the statement that the Universe looks the same from everywhere, and isotropy, ie. that it looks the same in every direction, seems a reasonable principle. In fact it is an assumption, called the Cosmological Principle.
In short distance scales, the Cosmological Principle is obviously false: the conditions (temperature, density etc) in the Sun, on Earth and in the region between Mars and Jupiter are obviously very different. Moreover the Universe seems to have structures, i.e. the planets rotate around stars, the stars cluster in Galaxies, and Galaxies cluster in clusters and superclusters. Superclusters and Voids are the largest known structures in the observable Universe right now. Superclusters have a size of 100Mly. In a scale of 300Mly the Universe looks pretty homogeneous. Note that our Galaxy is 0.1-0.2Mly in diameter, so if the typical scale where the Universe looks homogeneous is represented in a map of 3m length, our Galaxy would be a dot of size 1-2mm. Given the fact that the radius of the observable Universe is estimated to be 90.000Mly, this map would be 900m long.
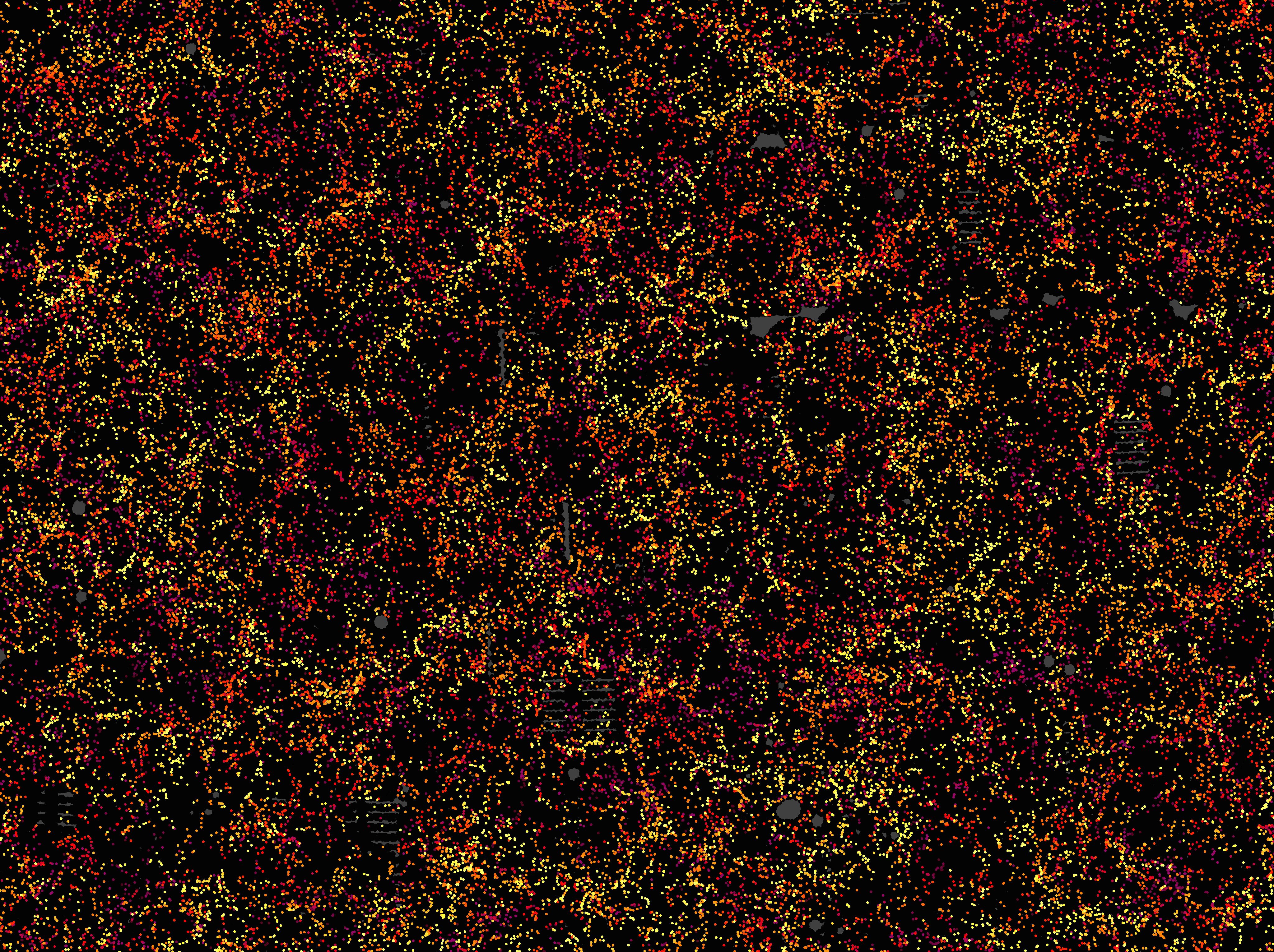
Isotropy is much more clearly measured by the CMB anisotropies, to be smaller than 10^(-5).
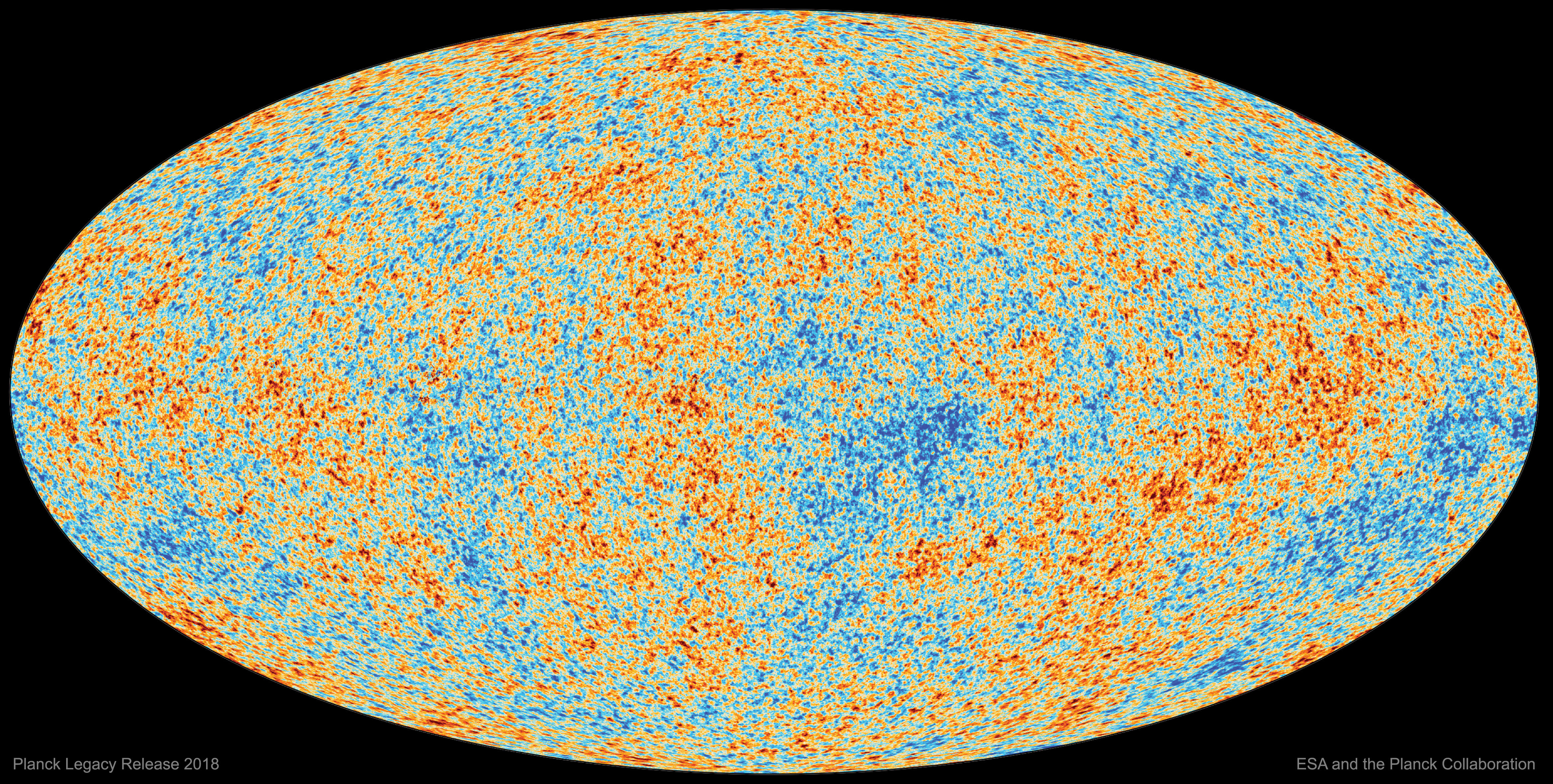
Hence, to a very good approximation, the Universe as a whole looks homogeneous and isotropic, as far as we can tell.
The Universe expands
This statement means that the distance between any two galaxies that are NOT gravitationally bound, increases with time. We know this because we observe light (spectral lines) from remote astronomical objects to be red-shifted.
In particular we observe light from a distant source as having a certain wavelength but we know (because the emission or absorption spectral line corresponds to a known atomic transition) that, in fact it was emitted at a smaller wavelength. We can define:
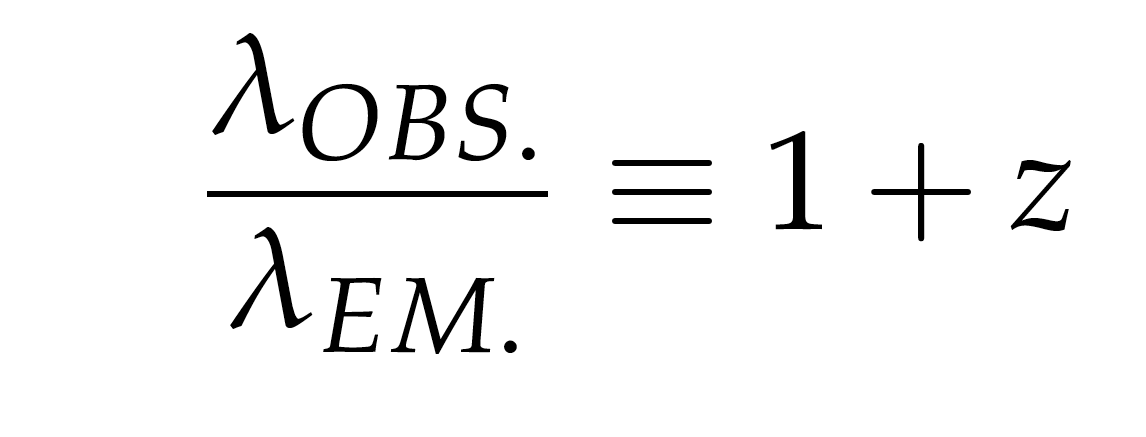
where z is called "the red-shift". For relatively nearby objects, which do not recede fast, the two wavelengths are practicly the same, and z is very close to 1.
It was found by Hubble that, for relatively small values of z, there is a linear relation between z and the distance:
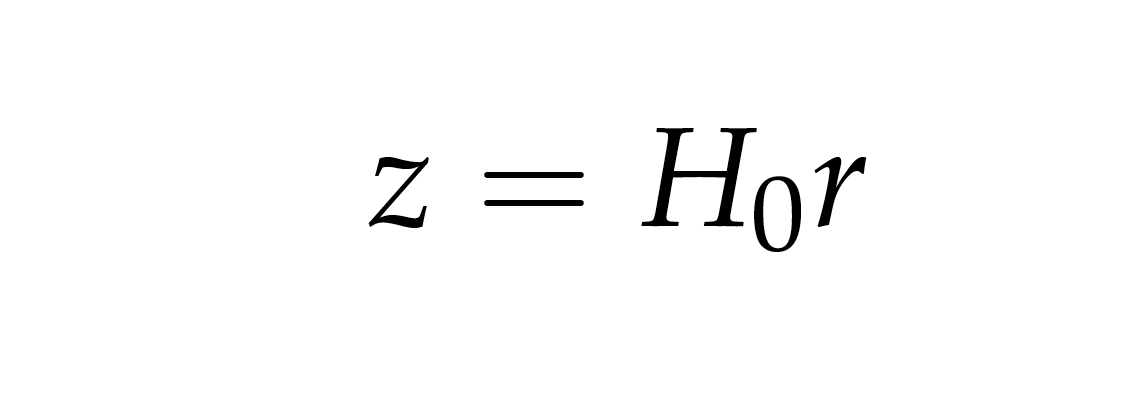
This was shocking: it implies that the further away an object is, the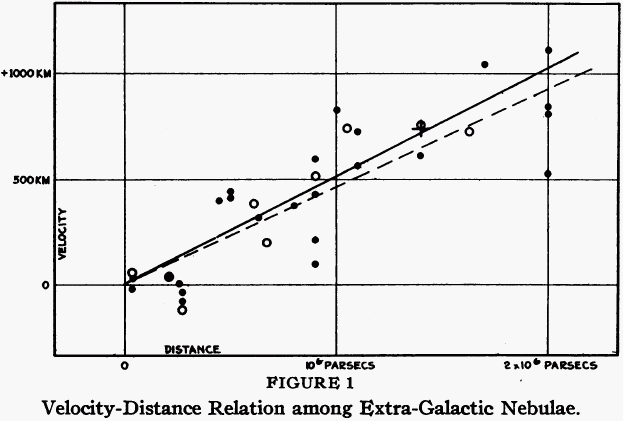 
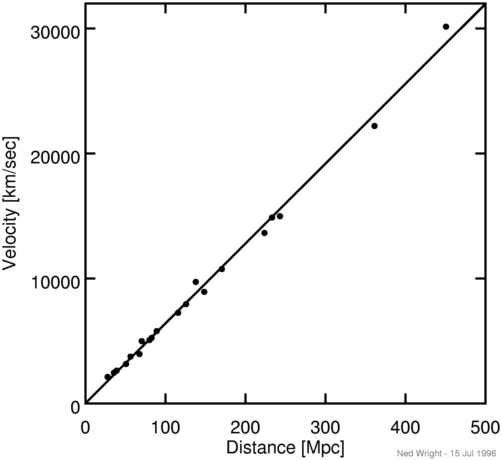For larger values of z, it is NOT, actually, a constant. We can define the Hubble
Estimating the distance to a remote object is done by comparing its luminosity (as opposed to the frequency of its light) with what the object would have if it would be nearby. Of course, in order to do this we need to observe so-called "Standard Candles", i.e. objects whose typical luminosity, if they were nearby, we (think we) would know. For example, historically, a type of giant or supergiant star called Cepheid played an important role in estimating distances. Cepheids have luminosities that correlate well with their rotation period. The rotation period itself results in the star pulsating, so it can be measured.
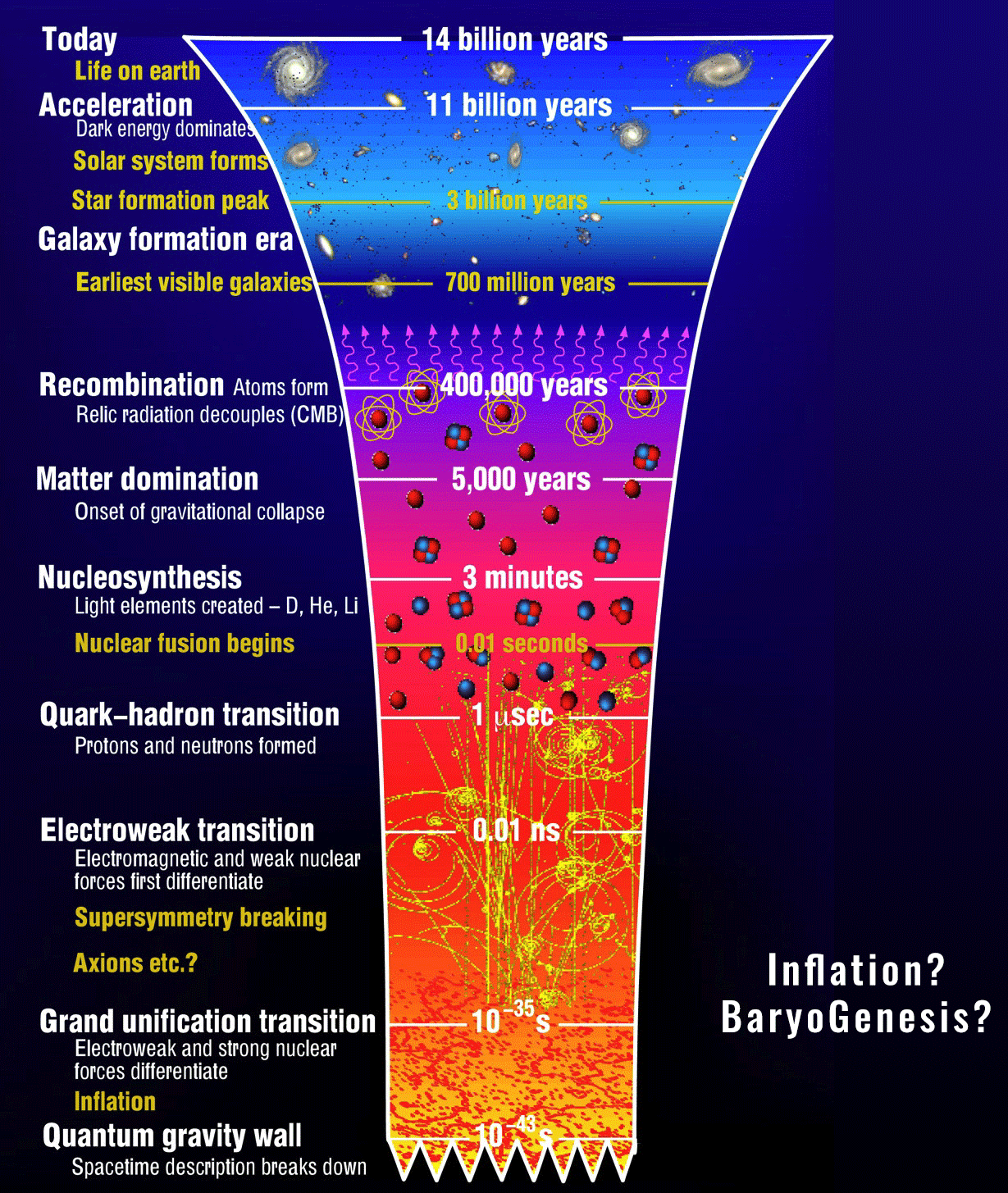
The Age of the Universe: 14 Billion Years
Various independent methods give results in the same ballpark. White dwarfs, for example, have a luminosity that decreases with time. Their abundance in the galaxy drops sharply below a certain luminosity threshold, indicating the age of the galaxy.
Another approach is to use the decaying processes of heavy nuclei as cosmic clocks. Measuring the relative abundance of two heavy nuclei today and taking their ratio can lead to an estimate of the time since their formation, if we know their relative abundance when they formed. But this depends on the conditions when they formed and they mechanism through which they form. For heavy nuclei like Uranium isotopes, we think we know the formation mechanism and the environment: such elements were formed within super-nova explosions by rapid neutron capture. This leads to an estimate of the relative abundance of two Uranium isotopes (the heavier one has more neutrons, so it’s rarer and we can compute how much). This, in turn leads to an estimate of the time since formation and if the super-novas were of first generation stars, a good minimum for the age of the Universe itself.
If we assume that our current theory for the Early Universe and its expansion is correct, we can also estimate the age of the Universe from the temperature of the Cosmic Background Radiation (see next section). This is a very precise estimate that leads to
13.799 +- 0.021By.
The Universe's expansion is Accelerating
At relatively small distances and therefore red-shifts, z less than 1, the Hubble law holds and the redshift is proportional to the distance from the Galaxy. At higher z, the light comes from further away, so it has emitted far in the past, and the relation between the redshift and the distance is influenced by whether the Universe expanded always in the rate that it does today, or not. Observing type Ia supernova it was established that the Universe expanded slower in the past than it does today, therefore the expansion rate is increasing, i.e. the Universe is in an accelerated expansion. The measurements were first performed for what is by today’s standards medium z, 0.16 - 0.97 but also verified at z larger than 1.25.
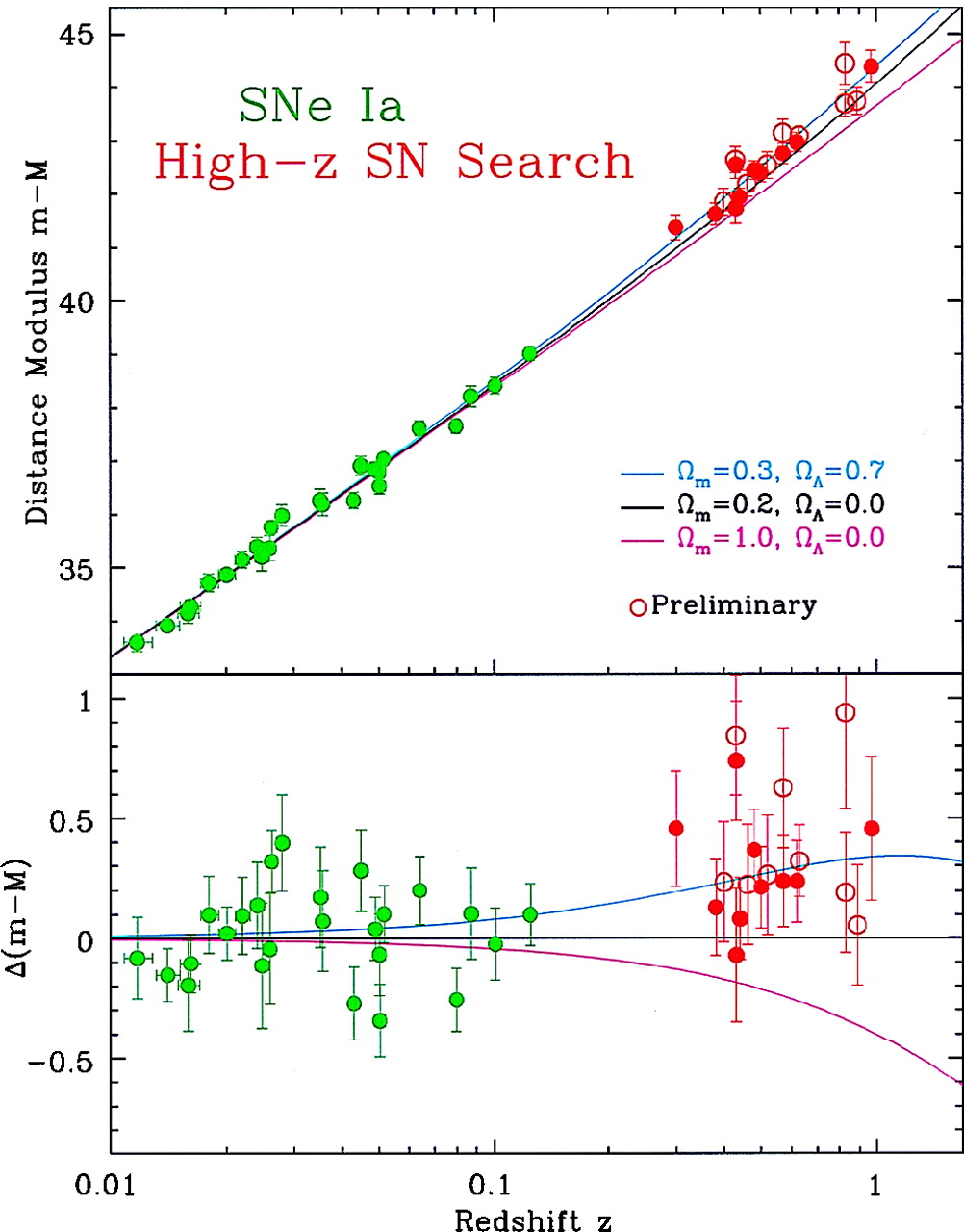
The accelerated exoansion of the Universe implies the existense of some substance responsible for it. We know almost nothing about this substance. We call it "dark energy".
Mass-Energy density
As we will see, General Relativity postulates an interplay between the content of the Universe (i.e. its energy density) and the geometry of space-time. If the energy density (which is a function of time, since the Universe expands) has a particular critical value at any given moment (e.g. today) then the Universe is exactly flat.
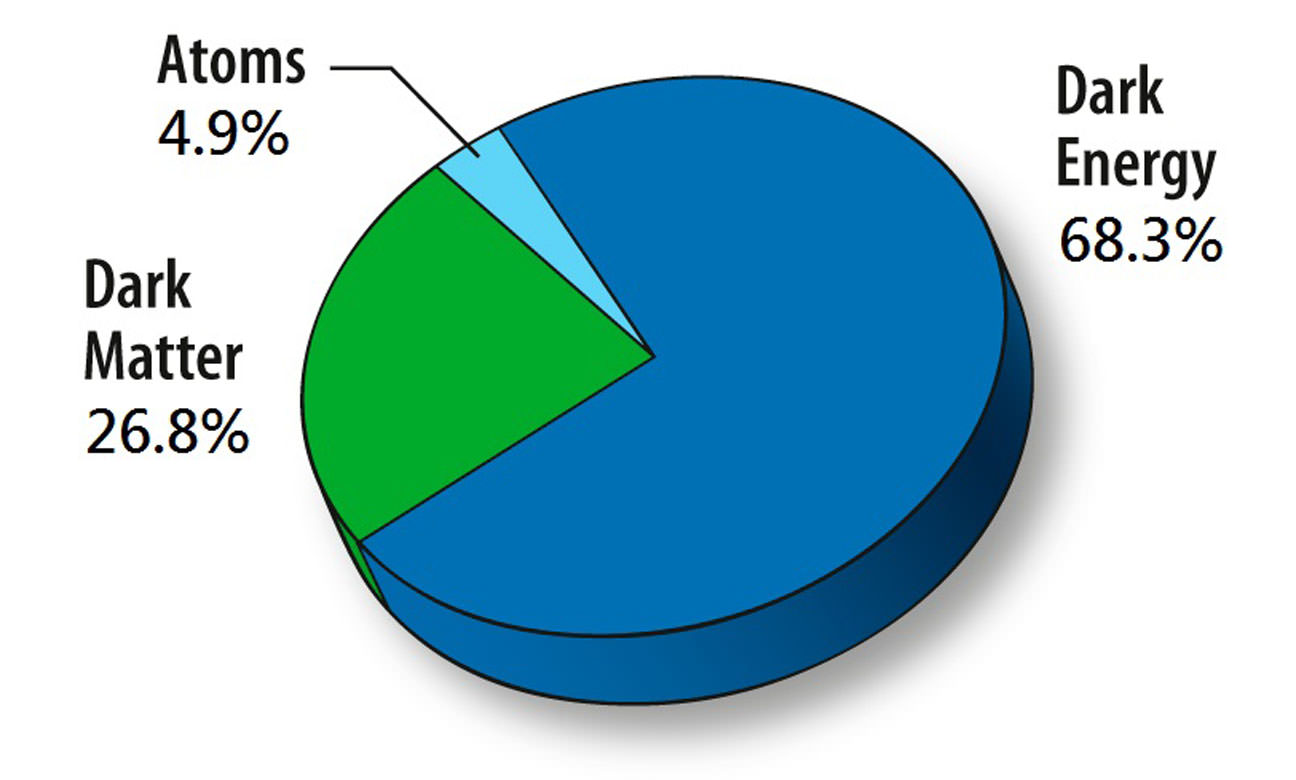
There are three possible contributions to the total energy density of the Universe: mass (either in the form of baryons or dark), radiation and "dark energy". As the Universe expands, the energy density of radiation drops much faster than the energy density of matter. As a result, today, the radiation (in the form of photons or relativistic particles, see later for definitions) contributes a negligible ammount to the total energy density.
If we measure, independently, the energy density due to matter(done in various ways), and the energy density due to dark energy (measuring the rate of acceleration), we find that the sum is equal to the critical density, within the margin of error. Hence our Universe seems flat.
Dark Matter
There are various ways to measure the mass density of the Universe that is due to baryons, i.e. matter as we know it around us. One way is to measure typical mass over luminosity ratios of close by objects, i.e. galaxies. Using this ratio and measuring the total luminosity of the sky, we get an estimate of the total mass of the visible Universe, and from its size, we deduce the baryonic density.
Another way to do this is to measure the abundance of the light elements in the Universe, i.e. those elements like Hydrogen, Deuterium, Helium and Lithium, that are not produced in stars. In particular, the abundance of Deuterium is determined by the amount of baryonic matter that existed in the Universe during the process of nucleosynthesis, in the first three minutes.
A third way to measure the baryonic density, and by now the most precise, is by studying the anisotropies of the Cosmic Microwave Background.
All these approaches point to a ratio of baryonic to non-baryonic matter being very close to 1/6. This non-baryonic matter is what we call "Dark Matter": it does not emmit light, does not interact with the baryonic matter significantly, apart from gravitation, and also does not self-interact much. It is also non-relativistic, hence we call it
The Grand Narrative
The Cosmological Principle, combined with the assumption that General Relativity is the correct theory of gravity at all scales, and the observation that the Universe expands, set up the framework of modern cosmology. We imagine the Universe as an expanding soup, from some initial, inaccessible to us phase, towards the current observable Universe.
As the Universe expands, the energy density of its components drop, and therefore the average energy per particle also drops (that's what we'll call the temperature of the particle species). When this average energy crosses various thresholds, reactions that used to be as abundant as their inverses, stop being possible. This disturbs the thermal equilibrium, and the Universe changes consistency. There are various such events in the history of the Universe. Each of them is a main theme for one or more lectures in the course. Here is a rough chronology of what we think today were the main events.
